The area of a triangle can be calculated with the following formula:
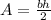
Where "b" is the base and "h" is the height of the triangle.
In this case, you can notice that:
![\begin{gathered} b=7.8\operatorname{cm} \\ h=5.78\operatorname{cm} \end{gathered}]()
In order to estimate the area of the triangle, you can follow these steps:
1. Round the base and the height to the nearest Whole number:
- For the base: since the digit after the Decimal point is greater than 5, you must round up.
- For the height: since the digit after the Decimal point is greater than 5, you must round up.
Then:
![\begin{gathered} b\approx8\operatorname{cm} \\ h\approx6\operatorname{cm} \end{gathered}]()
2. Then, substituting these values into the formula and evaluating, you get:
![A\approx\frac{(8\operatorname{cm})(6\operatorname{cm})}{2}\approx\frac{48\operatorname{cm}}{2}\approx24\operatorname{cm}^2]()
Therefore, the answer is: Option C.