Question

Consider the function f(x) -2x^3 + 36x^2 - 162x + 2f(x) has an inflection point at x = Cwhere C is ____

Answer 1

We will have the following:

First, we are given:

`f(x)=-2x^3+36x^2-162x+2`

Now, we determine the critical points:

`\begin{gathered} f^(\prime)(x)=-6x^2+72x-162=0 \\ \\ \Rightarrow x=(-(72)\pm√((72)^2-4(-6)(-162)))/(2(-6))\Rightarrow \\ x=9 \\ x=3 \end{gathered}`

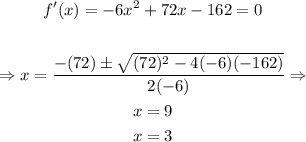
So, we can see that the expression has two critical points at x = 3 & x = 9.

So, at the points:

`(3,-214)`

&

`(9,2)`

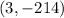
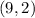
Now, we determine which one is the inflection point as follows:

`f^(\prime)^(\prime)(x)=-12x+72`

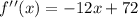
So, we analyze the function at the points given:

*x = 3:

`f^(\prime\prime)(3)=-12(3)+72\Rightarrow f^(\prime\prime)(3)=36`

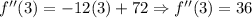
So, at x there is a local minimum.

*x = 9:

`f^(\prime)^(\prime)(9)=-12(9)+72\Rightarrow f^(\prime)^(\prime)(8)=-36`

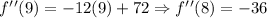
So, at x = 9 there is a local maximum.

The expression given has no inflection point.