STEP - BY - STEP EXPLANATION
What to find?
Domain
Graph of the function.
Given:
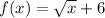
To solve the given problem, we will follow the steps below:
Step 1
Define the domain of a function
The domain of a function are set of input values for which the function is defined and real.
Step 2
Identify the domain.
In the given function, the function is not real for all set of negative numbers.
Hence, the domain of the function in interval notation is [ 0, ∞)
Step 3
Find the values of y at x=0, 1 and 2
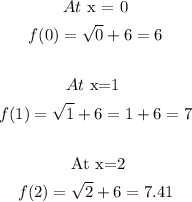
Step 4
Use the above result to form a table.
Step 5
Sketch the graph.
Step 6
Use the graph to determine the range.
The range is the set of values that correspond with the domain.
Hence, the range is [6, ∞)
ANSWER
• Domain : [ 0, ∞)
• The correct option is the, first graph,.
• ,Range, : , [6,, ,∞)