
Step-by-step explanation
when you have the slope and a point of the line, you can find the equation by using Point-slope is the general form
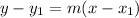
where
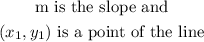
so
Step 1
Let
slope=-4/3
point=(-4,2)
replace and isolate y
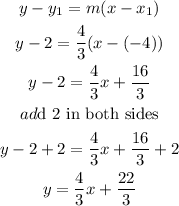
so, the equation of the line is
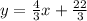
Step 2
plot 3 points
to do that, replace in the equation and you will get the y -coordinate
so
a) when x=0
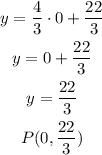
b) when x= 1
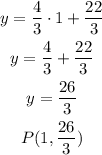
c) when x= -1
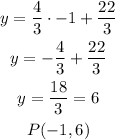
Step 3
finally, draw a line that passes through the points
I hope this helps you