Part A.
Since she will earn an additional $8,000 per year, then after 40 years she will have

So, the total amount of the additional earning is $320,000
Part B.
In this case, we need to use the compound interest formula, given by
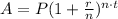
where A is the future value, P is the present value, r is the annual interest rate, n is the number of compounding periods per year and t is the time. In our case,
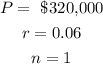
Then, by substituting these values into the formula, we have