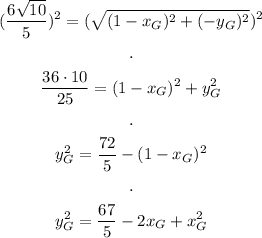The perimeter of a figure is the sum of the length of all its sides.
In the triangle ACE, we don't know the lengths of the sides, but we do know the coordinates of the points A, C, and E in the cartesian plane.
Given two points P and Q, we can find the distance between them using:
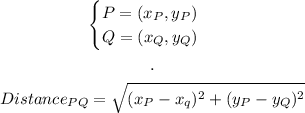
In this case, we have the points:
A = (3, 6)
C = (7, 2)
E = (1, 0)
Then we need to find the distances: AE, EC, and AC. Using the formula for distance:
AE:
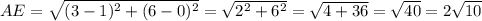
EC:
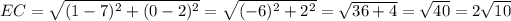
AC:
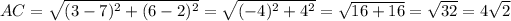
Now, we know the lengths of all the sides of the triangle. To find the perimeter, we need to add them:
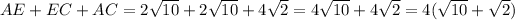
a) The perimeter is: 4(√10 + √2) units.
Now, to find the coordinates of point G, let's make a diagram:
I rotated the triangle so it lays over the base AE, indicated in a.
We have divided the triangle into two separate right triangles. We'll use the pythagorean theorem to solve this.
For triangle AGC:
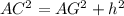
For triangle CGE:
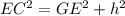
Now, we can note:
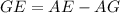
Using this in the equation for the triangle CGE:

We have the two equations:
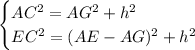
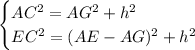
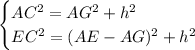
We know the values of AC, EC and AE. Then:

Now simplify both equations and solve for h².
Triangle AGC:
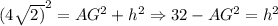
Triangle CGE:

We have two equations:

Now, we can equate both equations:

And solve:
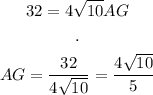
We know the distance between point A and point G.
We can use to find the distance between point E and point G:
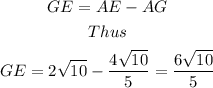
Now, we can use the formula for distance between two points, to create a system of equations.
Let be G:
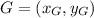
Distance between points A and G:
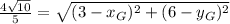
Distance between points E and G:
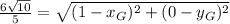
We have the system of euqations:
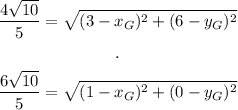
Let's take the first equation and simplify it:

Now the second equation: