Step-by-step explanation:
For any right triangle
the relationship between the hypotenuse and the adjacent side of an angle theta is the cosine of the angle:
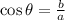
In this problem we have b = 16m and a = 25m. Therefore the cosine of x is:
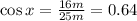
Using the inverse of the cosine - "the arc whose cosine is x" - we find x:
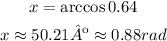
Answer:
The value of the angle is x = 50.21º or x = 0.88 radians