Question

A jury pool has 17 people that are married and 17 people that are not married, from which 12 jurors will be selected. Assuming that each person is equally likely to be chosen and that the jury is selected at random without replacement, find the probability that the jury consists of the following. (Give answer as a fraction or a decimal out to at least 4 places. If your answer is very small use scientific notation out to 4 decimal places for example 3.3421E-6.)(c) 8 married and 4 that are not married. (d) 6 married and 6 that are not married.

Answer 1

We are given the following information

Number of people that are married = 17

Number of people that are not married = 17

Total number of people = 17+17 = 34

Number of jurors to be selected = 12

We are asked to find the following probabilities.

(c) 8 married and 4 that are not married.

The total number of ways to select 12 jurors from a total of 34 people is given by

`^nC_r=(n!)/(r!(n-r)!)`

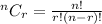
We have n = 34 and r = 12

`^(34)C_(12)=(34!)/(12!(34-12)!)=548,354,040`

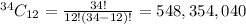
So, there are 548,354,040 ways to select 12 jurors from a total of 34 people.

The number of ways to select 8 married jurors from a total of 17 married people is given by

n = 17 and r = 8

`^(17)C_8=(17!)/(8!(17-8)!)=24,310`

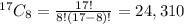
So, there are 24,310 ways to select 8 married jurors from a total of 17 married people.

The number of ways to select 4 not married jurors from a total of 17 not married people is given by

n = 17 and r = 4

`^(17)C_4=(17!)/(4!(17-4)!)=2,380`

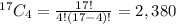
So, there are 2,380 ways to select 4 not married jurors from a total of 17 not married people.

Finally, the probability is

`P(8\; married\; and\; 4\; not\; married)=(^(17)C_8*^(17)C_4)/(^(34)C_(12))=(24,310*2,380)/(548,354,040)=(57,857,800)/(548,354,040)=0.1055`

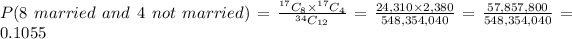
There is a 0.1055 probability that the jury consists of 8 married and 4 not married people.

(d) 6 married and 6 that are not married.

We have already found the total number of ways to select 12 jurors from a total of 34 people that is 548,354,040

The number of ways to select 6 married jurors from a total of 17 married people is given by

n = 17 and r = 6

`^(17)C_6=(17!)/(6!(17-6)!)=12,376`

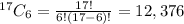
So, there are 12,376 ways to select 6 married jurors from a total of 17 married people.

The number of ways to select 6 not married jurors from a total of 17 not married people will also be the same that is 12,376

Finally, the probability is

`P(6\; married\; and\; 6\; not\; married)=(^(17)C_6*^(17)C_6)/(^(34)C_(12))=(12,376*12,376)/(548,354,040)=(153,165,376)/(548,354,040)=0.2793`

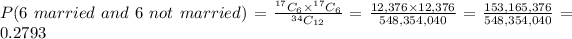
There is a 0.2793 probability that the jury consists of 6 married and 6 not married people.