Answer:
(a)14 seats
(b)1,050 seats
Step-by-step explanation:
The theater has 10 seats in the first row and 30 seats in the 6th row.
We can model this as an arithmetic progression problem where:
• The first term, a = 10
,
• The last term, l = 30 when n=6
We know that for an arithmetic progression:
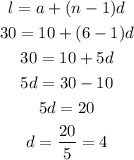
Therefore, the number of seats in the 11th row will be:
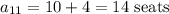
(b)The theater has a total of 21 rows.
To determine the total number of seats, we use the formula for the sum of an arithmetic progression.
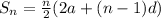
We define the variables:
• Since the theatre has a total of 21 rows, therefore n=21
,
• The first term, a = 10
,
• Common difference, d = 4
We substitute into the formula above:
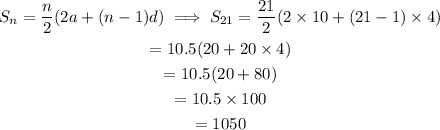
There are a total of 1,050 seats in the theater.