Question

mary purchased 4 tires by mail order. She paid $62.00 per tire plus $4.10 per tire for shipping and handling. there is no sales tax because the tires were purchased out of state. she also had to pay $7.11 per tire for mounting and balancing. at a local store , Mary's total for the 4 tires with mounting and balancing would be $328 plus 8% sales tax. how much did mary save by purchasing the tires through the mail?

Answer 1

Each tire costs $62.00 plus $4.10 for shipping and handling.

That is

`62+4.10=66.10`

$66.10 per tire, if she bought 4 tires, to determine the total cost for the tires + shipping you have to multiply this cost by 4

`66.10\cdot4=264.4`

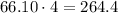
The total cost for the 4 tires + shipment fee is $264.40

Now she had to pay $7.11 per tire for mounting and balancing, multiply the price per tire by 4 to determine the cost for mounting and balancing the 4 tires:

`7.11\cdot4=28.44`

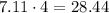
Mounting and balancing the 4 tires costs $28.44

The total cost for buying the tires out of state plus mounting and balancing them is

`264.40+28.44=292.84`

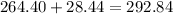
She paid a total price of $292.84 for b