1 Answer

Walves · Answer 1 · 2023-01-14T16:59:00+0000

Recall that the equation of a line in standard form is as follows:

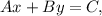
where A, B, and C are integers and A>0.

Now, to answer this question we will use the following slope-point formula for the equation of a line:

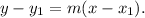
$y-y_1=m(x-x_1)\text{.}$

Therefore the equation of the line that passes through (-3,-6) and has a slope of 16/7 is:

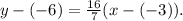
$y-(-6)=(16)/(7)(x-(-3))\text{.}$

Simplifying the above result we get:

$y+6=(16)/(7)(x+3)\text{.}$

Multiplying the above equation by 7 we get:

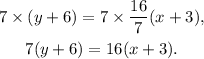
$\begin{gathered} 7*(y+6)=7*(16)/(7)(x+3), \\ 7(y+6)=16(x+3)\text{.} \end{gathered}$

Applying the distributive property we get:

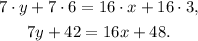
$\begin{gathered} 7\cdot y+7\cdot6=16\cdot x+16\cdot3, \\ 7y+42=16x+48. \end{gathered}$

Subtracting 7y from the above equation we get:

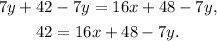
$\begin{gathered} 7y+42-7y=16x+48-7y, \\ 42=16x+48-7y\text{.} \end{gathered}$

Subtracting 48 from the above equation we get:

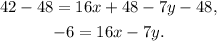
$\begin{gathered} 42-48=16x+48-7y-48, \\ -6=16x-7y\text{.} \end{gathered}$

Answer:

Please log in or register to add a comment.