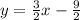Answer:
The equation of the line perpendicular to the given line is
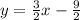
Step-by-step explanation:
Given the equation:
2x + 3y = 12
Let us rewrite it in standard form to have the slope and y-intercept.
Subtract 2x from both sides
3y = 12 - 2x
Divide both sides by 3
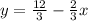
or
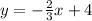
Here, the slope is -2/3, and the y-intercept is 4
An line perpendicular to this line has it's slope as the negative reciprocal of the slope -2/3
The negative reciprocal of -2/3 is 3/2
The perpendicular line is in the form:
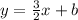
Where b is the y-intercept.
Since the line contains the point (-5, -12), -5 and -12 are the coordinates of the x and y axes respectively, using them, we can obtain a value for b, the y-intercept.
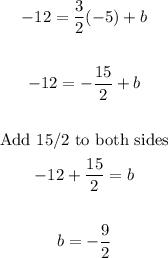
Therefore, the equation of the line perpendicular to the given line is