Question

Mark pushes his broken car 170 m down the block to his friend's house. He has to exert a 150 N horizontal force to push the car at a constant speed.How much thermal energy is created in the tires and road during this short trip?

Answer 1

25,500 J

Explanation:

First, we know that because the car is moving at a constant speed, the force that causes the thermal energy (friction) must be equal to the push force. So,
`F_(fr) = F_(push) = 150 N`

In this case, the thermal energy between the tires & the road and the work are equal to each other because the thermal energy from kinetic motion is the only energy present.

Therefore,

W =
`K_(e)`

The equation for work is
`W = fdcos\theta`

Plugging in W for
`K_(e)`:

`K_(e) = f_(fr) dcos\theta = (150)(170)(cos(180)) = - 25,500 J`

However, because it is asking for how much thermal energy is created, the value is made positive

+25,500 J

Answer 2

- The car moves at constant speed. This implies that the force on the car is 0N.

- If the resultant force on the car is 0N, it means that all the forces acting on the car add up to 0N.

- As a result, the amount of energy is conserved in the system.

- Thus, we can simply assume that all the energy put into pushing the car is converted to thermal energy via frictional forces.

- That is, we have:

`\begin{gathered} \vec{F}-\vec{f}=0N \\ \\ W=E_(th) \\ \\ where, \\ \vec{F}\text{ is the force applied in pushing the car} \\ \vec{f}\text{ is the frictional force of the car's tyres and the ground} \\ W\text{ is the work done on the car by pushing it} \\ E_(th)\text{ is the energy converted to thermal energy} \end{gathered}`

- Thus, if we can find the work done in pushing the car, we can automatically find the thermal energy according to the second formula.

- Thus, we have:

`\begin{gathered} W=\vec{f}*\vec{d} \\ W=150*170 \\ W=25,500J \\ \\ \text{ Thus,} \\ E_(th)=25,500J \end{gathered}`

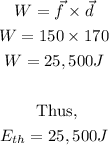
Final Answer

The thermal energy is 25,500J