Question

Suppose that y varies inversely as the square of x, and that y = 8 when x = 17. What is y when x = 14? Round your answer to two decimal places if necessary.

Answer 1

When two variables are inversely proportional we can represent them in the following manner:

`y\text{ = }(a)/(x)`

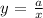
Where "a" would be the constant of proportionality. In the case of our problem the y is inversely proportional to the square of "x", this means that the correct expression is:

`y=(a)/(x^2)`

We need to find the value of "a", to do that we will apply the ordered pair which was given to us (17,8).

`\begin{gathered} 8=(a)/((17)^2) \\ a=8\cdot(17)^2 \\ a=8\cdot289 \\ a=2312 \end{gathered}`

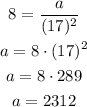
Therefore the expression to this problem is:

`y=(2312)/(x^2)`

We want to find the value of "y" when "x" is equal to 14, therefore:

`\begin{gathered} y=(2312)/((14)^2) \\ y=(2312)/(196) \\ y=11.8 \end{gathered}`

The value of "y" is 11.8