Solution
- The box must hold the same shape as the pyramix and be tight around it. This means that the box must have a surface area equal to that of the pyramix.
- This simply means we need to find the surface area of the pyramid shape of the pyramix.
- To do this, we simply need to find the area of one triangular face and multiply by 4 since there are 4 faces.
- The area of an equilateral triangle is:
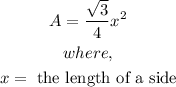
- We know the length of a triangle in the pyramid is 3.75, thus, the amount of material needed to cover each of the 4 sides is:
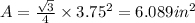
- Each of the 4 sides need to be covered by 6.089 square inches of material