Question

Help with word problems please help me with both of them :)

Answer 1

Given:

Christopher has 20 video games and will buy 4 more each month

Peter has 92 video games and will sell 5 each month

Let the number of months required for them to have an equal number of video games be x

Hence:

`\begin{gathered} 20\text{ + 4}*\text{ x = 92 - 5}* x \\ 20\text{ + 4x = 92 -5x} \end{gathered}`

Solving for x:

`\begin{gathered} \text{Collect like terms} \\ 4x\text{ + 5x = 92 - 20} \\ 9x\text{ = 72} \\ \text{Divide both sides by 9} \\ (9x)/(9)\text{ = }(72)/(9) \\ \text{x = 8} \end{gathered}`

The number of video games they would both have is obtained by substitution

`\begin{gathered} =\text{ 20 + 4x} \\ =\text{ 20 + 4}*8 \\ =\text{ 20 + 32} \\ =\text{ 52} \end{gathered}`

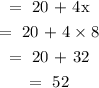
Answer:

8 months , 52 video games