Question

A boat travels downstream 10 miles and returns to the starting point in 2 hours. If the speed of the stream is 15 mph, find the speed of the boat in still water.

Answer 1

Let's call the speed of the boat in still water as x.

One part of the trip is 10 miles downstream, where the speed of the boat and the speed of the current add together and the other part of the trip is the trip back the 10 miles upstream, where the speed of the current works against the speed of the boat, which gives to us the following equation:

`(10)/(x+15)+(10)/(x-15)=2`

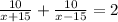
Solving for x, we have:

`\begin{gathered} (10)/(x+15)+(10)/(x-15)=2 \\ 10(x-15)+10(x+15)=2(x-15)(x+15) \\ 10x-150+10x+150=2(x^2-15^2) \\ 20x=2(x^2-225) \\ 10x=x^2-225 \\ x^2-10x-225=0 \end{gathered}`

Using the quadratic equation, the solution for our equation is:

`x\approx20.81`

The answer is 20.81 mph.