Question

Maggie and three friends are going to the movies. It cost them twelve dollars for theirtickets and snacks cost two dollars each. In interval form, how many snacks can they buy ifthey brought twenty dollars?

Answer 1

Given:

The cost of tickets, x=$12.

The total number of people gone for movie, n=4.

The cost of each snack, y=$2.

The total amount brought by the friends, T=20.

Let m be the maximum number of snacks bought.

Now, the expression for the total amount if all the money is spent is,

`\begin{gathered} T=x+ym \\ 20=12+2m \end{gathered}`

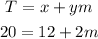
Solve the above expression for m.

`\begin{gathered} 2m=20-12 \\ 2m=8 \\ m=(8)/(2) \\ m=4 \end{gathered}`

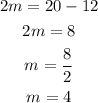
So, the maximum number of snacks they can buy is 4.

Therefore, they can buy 0, 1, 2, 3 or 4 number of snacks.

In interval notation, the number of snacks they can buy is [0, 4].