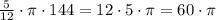The formula for the total area of a circle is the following:
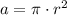
where r is the radius of the circle (12 in this case).
The total area of this circle then is
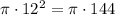
The circle in total has 360 degrees, of which you only want the area that 150 degrees of this circle. To do this, you must find the percentage of the circle you want to find, like this:
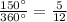
Then, the area of the sector you must find is the total area by the percentage of that area: