Question

3 out of 20 cars passing through an intersection did not fully stop, what is the probability that a car arriving at this intersection will not fully stop

Answer 1

Step 1: State the given in the question

Given that 3 out 20 cars passing through an intersection did not fully stop

Step 2: State what to be determined

We are to determine the probability that a car arriving at this intersection that will not fully stop

Step 3: State the formula for finding probability

The formula for finding the probability of an en event, E, from a sample S, is the ratio of the number of elements of event E to the total number of elements in the sample S.

This can be represented mathematically as

`\begin{gathered} P(E)=(n(E))/(n(S)) \\ \text{Where} \\ P(E)=\text{Probability of an event E occuring} \\ n(E)=\text{Number of element in event E} \\ n(S)=\text{Total number of elements in the sample} \end{gathered}`

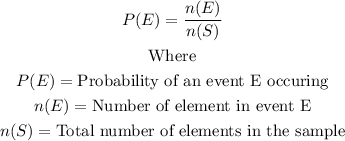
Step 4: Use the formula to solve the probability

If the event E is cars passing through an intersection did not fully stop. Then, the number of elements in the event E is given as 3. That is:

`n(E)=3`

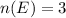
The sample is the total number of cars, which is given as 20. This means that

`n(S)=20`

Therefore, the probability of the event occuring would be P(E). This is as calculated below:

`\begin{gathered} P(E)=(n(E))/(n(S)) \\ n(E)=3 \\ n(S)=20 \\ P(E)=(3)/(20) \\ P(E)=0.15 \end{gathered}`

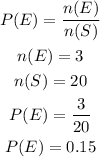
Hence, the probability that a car arriving at this intersection will not fully stop is 3/20 or 0.15