Question

The hypotenuse of a right triangle is four times the length of one of the legs. The length of the other leg is sqrt(240) feet. Find the lengths of the leg and hypotenuse

Answer 1

Hypotenuse: 16 ft

Leg: 4 ft

Step-by-step explanation

Given:

A right angle triangle with only one leg = sqrt(240) feet

Desired Outcomes:

Lengths of the leg and hypotenuse

Declaration of variables

Let x represent the length of leg

Hypotenuse = 4 times the length of leg = 4x

Apply Pythagorean theorem

`\begin{gathered} Hyp^2=Opp^2+Adj^2 \\ (4x)^2=x^2+(√(240))^2 \\ 16x^2\text{ = }x^2\text{ + 240} \\ 16x^2-x^2\text{ = 240} \\ 15x^2\text{ = 240} \\ x^2\text{ = }(240)/(15) \\ x\text{ =}√(16) \\ x\text{ = 4 ft} \end{gathered}`

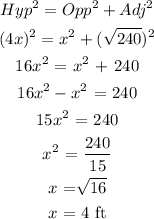
Hypotenuse = 4x = 4 (4) = 16 ft

Hence, the Lengths of the leg and hypotenuse are 4 ft and 16 ft respectively.