Step-by-step explanation
let
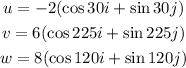
Step 1
Part A)Find −7u • v.
the dot product is the sum of the products of the corresponding entries of the two sequences of numbers,and to multiply a vector by a scalar, multiply each component by the scalar.
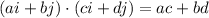
so
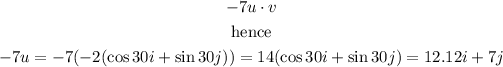
therefore, replacing
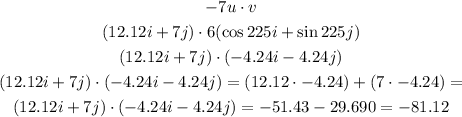
So,Part A
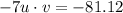
Step 2
Part B ,Use the dot product to determine if u and w are parallel, orthogonal, or neither
the dot product is also given by:
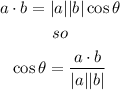
a) find the magnitude of the vectors
![\begin{gathered} \text{ }u=-2\mleft(\cos 30\degree i+\sin 30\degree j\mright), \\ u=(-2\cos 30\degree i-2\sin 30\degree j) \\ \text{hence} \\ \lvert u\rvert=\sqrt[]{(-2\cos30)^2+(-2\sin30\degree)}^2 \\ \lvert u\rvert=\sqrt[]{3^{}+1\text{ }} \\ \lvert u\rvert=\sqrt[]{4}=2 \\ \lvert u\rvert=2 \end{gathered}](https://img.qammunity.org/2023/formulas/physics/college/por0bzgwkvc016oextrjf55izpxq2o5sck.png)
and
![\begin{gathered} \text{ w}=8\mleft(\cos 120\degree i+\sin 120\degree j\mright) \\ w=8(\cos 120\degree i+\sin 120\degree j)=-4i+6.92j \\ \text{hence} \\ \lvert w\rvert=\sqrt[]{(-4)^2+(6.92)^2} \\ \lvert w\rvert=\sqrt[]{16^{}+48} \\ \lvert w\rvert=\sqrt[]{64} \\ \lvert w\rvert=8 \end{gathered}](https://img.qammunity.org/2023/formulas/physics/college/qguvise7avvux0negpmu0mj42t93k4imql.png)
c) dot product
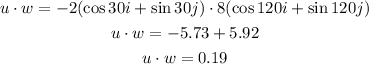
finally, replace in the formula and find the angle
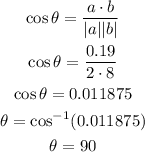
so
the vectors are orthogonal ( make a 90° angle)
I hope this helps you