The first step to solve this question is to state the neutralization reaction between KOH and HClO:
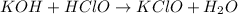
Now, find the amount of moles of HClO present in 50mL of 0.170M HClO:
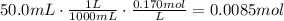
According to the equation, 1 mole of KOH reacts with 1 mole of HClO. Use this ratio to find the amount of moles of KOH that react with 0.0085moles of HClO:

Multiply this amount of moles of KOH by the inverse of its conversation:

It means that 0.05L of 0.170M KOH are needed to neutralize 50mL of 0.170M HClO.