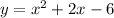Question

Find an equation in standard form of the parabola passing through the points below. (1,-3), (3,9), (4,18)

Answer 1

In standard form, the equation of a parabola is:

`ax^2+bx+c=y`

We have three points, and we evaluate this equation for these three points. For the point (1, -3):

`a+b+c=-3\ldots(1)`

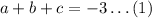
For (3, 9):

`9a+3b+c=9\ldots(2)`

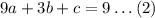
For (4, 18):

`16a+4b+c=18\ldots(3)`

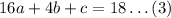
Now, subtracting (1) from (2) and (3):

`\begin{gathered} \begin{cases}8a+2b=12 \\ 15a+3b=21\end{cases} \\ \\ \Rightarrow\begin{cases}4a+b=6\ldots(4) \\ 5a+b=7\ldots(5)\end{cases} \end{gathered}`

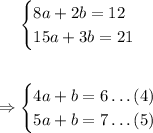
Solving this system of equations leads to ((5) - (4)):

`\begin{gathered} 5a+b-4a-b=7-6 \\ \Rightarrow a=1 \end{gathered}`

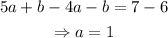
Now, using this result in (4):

`\begin{gathered} 4\cdot1+b=6 \\ \Rightarrow b=2 \end{gathered}`

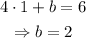
Finally, using a and b in (1):

`\begin{gathered} 1+2+c=-3 \\ \Rightarrow c=-6 \end{gathered}`

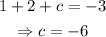
The standard form of the parabola passing through the three points (1,-3), (3,9), and (4,18) is:

`y=x^2+2x-6`