Small drink: 12 ounces
Large Drink: 36 ounces
Step-by-step explanation
Step 1
set the equations
let x represents the number of ounces of one small drink
let y represents the number of ounces of a large drink
hence
a)three small drinks and two large drinks contain 108 ounces of cola
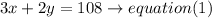
b)A small drink contains a third as much cola as a large drink
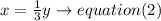
Step 2
solve the equations
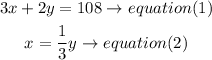
a) replace the x value from equation (2) into equation (1) and solve for y
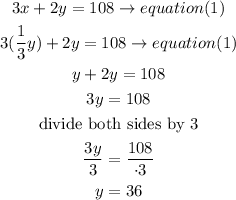
b) now, replace the y value into equaiton (2) to find the x value
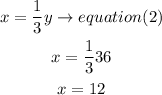
therefore:
Small drink: 12 ounces
Large Drink: 36 ounces
I hope this helps you