Answer:
5 mph
Explanation:
Let the rate (speed) of the boat in still water = x
The speed of the current = 3 mph
Thus:
• The speed against the current = (x-3) mph
,
• The speed with the current = (x+3) mph

Since the time it took on both trips is the same, we have that:
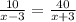
So, we solve the equation for x:
![\begin{gathered} \text{ Cross multiply} \\ 10\left(x+3\right)=40\left(x-3\right) \\ Open\text{ the bracket} \\ 10x+30=40x-120 \\ 30+120=40x-10x \\ 150=30x \\ \text{ Divide both sides by 30} \\ (150)/(30)=(30x)/(30) \\ x=5\;mph \end{gathered}]()
The rate of the boat in still water is 5 mph.