Question

4

An ice cream shop has a sign in the shape
of an ice cream cone. The sign is made
using a semicircle and a triangle, as
modeled below.
The base of the
triangle is the
6 in.
diameter of the
semicircle.
12 in.
Which is the best estimate of the area of
the sign in square inches?
F 150 in.2
F
G 14 in.2
H 36 in.2
J 50 in.2

Answer 1

In order to determine the total area of the sign, we need to calculate the area of the triangle, and the area of the semicircle. The expressions we need to use are described below:

`\begin{gathered} A_{\text{semicircle}}=(\pi\cdot r^2)/(2) \\ A_{\text{triangle}}=(b\cdot h)/(2) \end{gathered}`

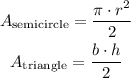
We were given the diameter of the semicircle, it's radius is half of the diameter, therefore:

`r=(6)/(2)=3\text{ in}`

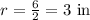
The base of the triangle is equal to the diameter of the semicircle. With this we can calculate both areas:

`\begin{gathered} A_{\text{triangle}}=(6\cdot12)/(2)=36\text{ square inches} \\ A_{\text{semicircle}}=(\pi\cdot3^2)/(2)=14.13\text{ square inches} \end{gathered}`

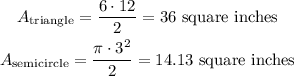
The total area of the figure is the sum of the above, therefore:

`\begin{gathered} A=A_{\text{triangle}}+A_{\text{semicircle}} \\ A=36+14.13=50.13 \end{gathered}`

The area is approximately 50 in². The correct option is J.