Question

Say a certain manufacturing industry has 63.1 thousand jobs in 2008, but is expected to decline at arate of 1.7 thousand jobs per year from 2008 to 2018. Assuming this holds true, what will be this induchange from 2008 to 2018?a. 70%b. -27%C. -17%d. -75%

Answer 1

The inicial number of jobs is 63.1 thousand, and each year this number will decrease by 1.7 thousand.

So the change from 2008 to 2018, that is, 10 years, will be:

`10\cdot(-1.7)=-17`

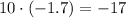
To find the change in percentage, we need to divide the change (-17) by the inicial amount of jobs (63.1), then we have that:

`(-17)/(63.1)=-0.27=-27\text{\%}`

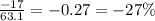
So the change in this period is -27%, therefore the answer is B.