Question

To determine the value of tangent of 7 times pi over 8, which identity could be used?

Answer 1

Given:

`\tan (7\pi)/(8)`

To Determine: The identity that is equivalent to the given tangent

Note that, the identity rule below would be applied

`\tan (\alpha)/(2)=\sqrt[]{(1-\cos \alpha)/(1+\cos \alpha)}`

Also,

`\tan (\alpha)/(2)=(\sin \alpha)/(1+\cos \alpha)`

And also,

`\tan (\alpha)/(2)=(1-\cos \alpha)/(\sin \alpha)`

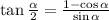
From the given tangent, we can re-write it as below:

`\begin{gathered} \tan (7\pi)/(8)\cong\tan ((7\pi)/(4))/(2) \\ \text{Note} \\ (7\pi)/(8)=((7\pi)/(4))/(2) \end{gathered}`

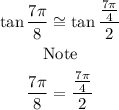
Therefore:

`\tan ((7\pi)/(4))/(2)=\sqrt[]{(1-\cos(7\pi)/(4))/(1+\cos(7\pi)/(4))}`

Also:

`\tan ((7\pi)/(4))/(2)=(\sin (7\pi)/(4))/(1+\cos (7\pi)/(4))`

And also,

`\tan ((7\pi)/(4))/(2)=(1-\cos (7\pi)/(4))/(\sin (7\pi)/(4))`

It can be observed from the option provided, the correct options is

I and III only