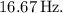The lowest frequency for standing waves on a long string with a wave speed of
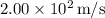 is approximately
is approximately
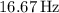 .
.
To determine the lowest possible frequency for standing waves on the 6.00-meter long string, we can use the formula:
![\[ f = (v)/(2L) \]](https://img.qammunity.org/2023/formulas/physics/college/hv7mzxc7744th6hwuw0snjx3a5vk3jw8fk.png)
where:
-
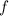 is the frequency,
is the frequency,
-
 is the wave speed,
is the wave speed,
-
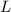 is the length of the string.
is the length of the string.
In this case, the wave speed
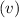 is given as
is given as
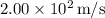 , and the length of the string
, and the length of the string
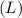 is 6.00-meters. Substituting these values into the formula:
is 6.00-meters. Substituting these values into the formula:
![\[ f = \frac{2.00 * 10^2 \, \text{m/s}}{2 * 6.00 \, \text{m}} \]](https://img.qammunity.org/2023/formulas/physics/college/6ehnlyv5a7emz44umidm09ebg4poft5ssp.png)
Simplifying the expression:
![\[ f = \frac{100 \, \text{Hz}}{6} \]](https://img.qammunity.org/2023/formulas/physics/college/koytuxkk37ui71nm78ayu9tyq49o84fcod.png)
![\[ f \approx 16.67 \, \text{Hz} \]](https://img.qammunity.org/2023/formulas/physics/college/nixyvlruubzcwdiiav7svnhshvm6hkwkku.png)
Therefore, the lowest possible frequency for standing waves on the 6.00-meter long string is approximately