Step-by-step explanation:
Step 1:
We will calculate the z-score using the formula below
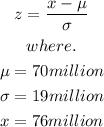
By substituting the values, we will have
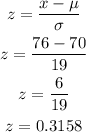
Step 2:
We will have to calculate the probabaility below
Using a p-valu calculat
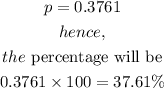
Hence,
The final answer to the nearest hundredth is