Question

A car traveling at 11.6 meters per second crashes into a barrier and stops in 0.287 meters. What force must be exerted on a child of mass 21.2 kilograms to stop him or her in the same time as the car? Answer must be in 3 significant digits.

Answer 1

The equation to obtain the final speed of car is,

`v^2=u^2+2as`

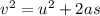
Substitute the known values,

`\begin{gathered} (0m/s)^2=(11.6m/s)^2+2a(0.287\text{ m)} \\ a=\frac{-134.56m^2s^(-2)}{2(0.287\text{ m)}} \\ \approx-234.4m/s^2 \end{gathered}`

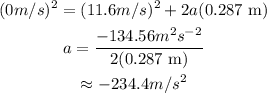
The negative sign of acceleration indicates that the car is deaccelerating.

The force required to stop the car is,

`F=ma`

Substitute the magnitude of known values,

`\begin{gathered} F=(21.2kg)(234.4m/s^2)(\frac{1\text{ N}}{1kgm/s^2}) \\ =4969.28\text{ N} \\ \approx4970\text{ N} \end{gathered}`

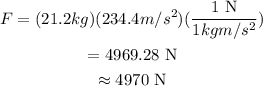
Thus, the force required to stop the car is 4970 N.