Question

Use function composition to verify f(x)=-3x+5 and g(x)=5x-3 are inverses. Type your simplified answers in descending powers of x an do not include any spaces between your characters.Type your answer for this composition without simplifying. Use parentheses to indicate when a distribution is needed to simplify. g(f(x))=AnswerNow simplify the composition, are f(x) and g(x) inverses? Answer

Answer 1

• (a)g[f(x)]=5(-3x+5)+5

,

• (b)No

Explanation:

Given f(x) and g(x):

`\begin{gathered} f(x)=-3x+5 \\ g(x)=5x-3 \end{gathered}`

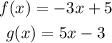
(a)First, we find the composition, g[f(x)].

`\begin{gathered} g(x)=5x-3 \\ \implies g\lbrack f(x)\rbrack=5f(x)-3 \\ g\lbrack f(x)\rbrack=5(-3x+5)+5 \end{gathered}`

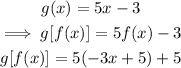
(b)Next, we simplify g[f(x)] obtained from part (a) above.

`\begin{gathered} g\mleft[f\mleft(x\mright)\mright]=5\mleft(-3x+5\mright)+5 \\ =-15x+25+5 \\ =-15x+30 \end{gathered}`

Given two functions, f(x) and g(x), in order for the functions to be inverses of one another, the following must hold: f[g(x)]=g[f(x)]=x.

Since g[f(x)] is not equal to x, the functions are not inverses of one another.