Question

I need help This is from my trig prep guide

Answer 1

From the question given, we have the following data;

Height of the tree = 80 feet

Angle of elevation to the top of the tree = 68 degrees

Distance from Corey to the tree = unknown

We shall now call the unknown variable x.

With that we shall have the following diagram;

We now have a diagram detailing the triangle and the dimensions showing Corey, the tree and the eagle at the tree top.

To get a better look, Corey moves several steps away from the tree and now determines his new angle of elevation to be 41 degrees.

This can now be illustrated as follows;

From triangle EDC, we shall calculate the distance from point C to point D using trigonometric ratios. The reference angle is at point C, which means the opposite side is side ED. The adjacent side is side CD (labeled x). Using trig ratios we have;

`\begin{gathered} \tan \theta=\frac{\text{opp}}{\text{adj}} \\ \tan 68=(80)/(x) \end{gathered}`

We cross multiply and we now have;

`\begin{gathered} x=(80)/(\tan 68) \\ U\sin g\text{ a calculator, we have tan 68 as 2.475086}\ldots \\ x=(80)/(2.475086) \\ x=32.322109\ldots \\ \text{Rounded to the nearest hundredth of a foot;} \\ x=32.32ft \end{gathered}`

Looking at triangle EDB;

The reference angle is 41 which makes the opposite side ED and the adjacent side BD. To calculate the distance BD, we'll have;

`\begin{gathered} \tan \theta=\frac{\text{opp}}{\text{adj}} \\ \tan 41=(80)/(BD) \\ We\text{ cross multiply and we now have;} \\ BD=(80)/(\tan 41) \\ BD=(80)/(0.869286) \\ BD=92.02955\ldots \\ \text{Rounded to the nearest hundredth;} \\ BD=92.03 \end{gathered}`

Take note that the distance Corey moved before he had a new angle of elevation is line segment CD which is indicated as y. Note also that

`\begin{gathered} BC+CD=BD \\ CD=x=32.32ft \\ BC+32.32=92.03 \\ \text{Subtract 32.32 from both sides;} \\ BC=59.71 \end{gathered}`

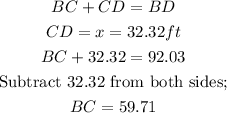
The distance Corey stepped back is indicated as y (line segment BC).

ANSWER:

Corey stepped back 59.71 feet