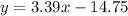Question

xyx2xy1645256720 2258 484 1,2762873 7842,044 3294 1,0243,008 45141 2,025 6,345 ∑x=143 ∑y=411 ∑x2=4,573 ∑xy=13,393 Which regression equation correctly models the data?y = 2.87x + 0.12y = 2.87x + 11.85y = 3.39x – 14.75y = 3.39x – 9.24

Answer 1

We are asked to identify the correct regression equation.

The regression equation is given by

`y=bx+a`

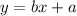
Where the coefficients a and b are

`a=(\sum y\cdot\sum x^2-\sum x\cdot\sum xy)/(n\cdot\sum x^2-(\sum x)^2)`
`b=(n\cdot\sum xy-\sum x\cdot\sum y)/(n\cdot\sum x^2-(\sum x)^2)`

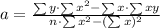
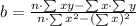
Where n is the number of observations that is 5.

Let us substitute the following into the above formula.

∑x=143

∑y=411

∑x^2=4,573

∑xy=13,393

`a=(411\cdot4573-143\cdot13393)/(5\cdot4573-(143)^2)=-14.75`
`b=(5\cdot13393-143\cdot411)/(5\cdot4573-(143)^2)=3.39`

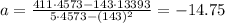
So, the coefficients are

a = -14.75

b = 3.39

Therefore, the correct regression equation is

`y=3.39x-14.75`