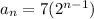Answer:
Step-by-step explanation:
Given the sequence with the recursive formula:
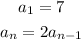
First, we determine the first three terms in the sequence.

Therefore, the first three terms of the sequence are: 7, 14 and 28.
This is a geometric sequence where:
• The first term, a=7
,
• The common ratio, r =14/7 = 2
We use the formula for the nth term of a GP.
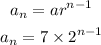
The explicit formula for the sequence is: