Answer:
56.25
Explanation:
We are told that the side lengths of the smaller figure are 3/4 the length of the larger figure.
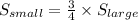
Now since the area is proportional to the equal of the side lengths, we have

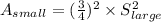
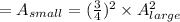
The last is true since A_large = S^2_large.
Now we are told that A_large = 100 square units; therefore,
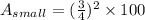
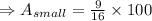
which we evaluate to get
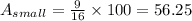
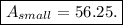
Hence, the area of the smaller figure is 56.25.