Let:
x = Cost of one plain pizza
y = Cost of one soda
two friends went to a restaurant and ordered one plain pizza and two sodas. the bill totaled $15.95. so:

later that day, five friends went to the same restaurant. they ordered 3 plain pizzas and 5 sodas. their bill totaled $45.90. so:

Let:
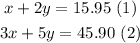
From (1) solve for x:
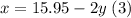
Replace (3) into (2):
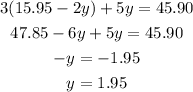
Replace the value of y into (3):
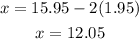
Therefore, the price of one plain pizza is $12.05