The numbers of regular-season wins for 10 football teams in a given season are given below

We are asked to find the range, mean, variance, and standard deviation of the population data set.
Range:
The range is the difference between the maximum value and the minimum value in a data set.
From the given data set,
Maximum value = 15
Minimum value = 2

Therefore, the range is 13
Mean:
The population mean is given by
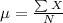
Where X is the terms in the data set and N is the number of terms in the data set.
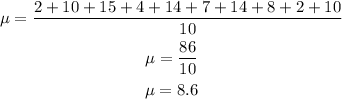
Therefore, the population mean is 8.6
Variance:
The population variance is given by
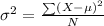
Where X is the terms in the data set, μ is the mean, and N is the number of terms in the data set.
![\begin{gathered} \sigma^2=\frac{\sum^{}_{}(X-\mu)^2}{N} \\ \sigma^2=((2-8.6)^2+(10-8.6)^2+(15-8.6)^2+(4-8.6)^2+(14-8.6)^2+(7-8.6)^2+(14-8.6)^2+(8-8.6)^2++(2-8.6)^2++(10-8.6)^2)/(10) \\ \sigma^2=(214.4)/(10) \\ \sigma^2=21.4 \end{gathered}]()
Therefore, the population variance is 21.4
Standard deviation:
The population standard deviation is given by
![\begin{gathered} \sigma^{}=\sqrt[]{\frac{\sum^{}_{}(X-\mu)^2}{N}} \\ \sigma=\sqrt[]{\sigma^2} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/3ijok21fmodjc2giiatss9l510bjtnenou.png)
Since we have already find the population variance, we can simply find take the square root of variance.
![\begin{gathered} \sigma=\sqrt[]{\sigma^2} \\ \sigma=\sqrt[]{21.4} \\ \sigma=4.6 \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/74i76o3oz3qeb4q46ko1so2uj0q0wx04dw.png)
Therefore, the population standard deviation is 4.6