Given that CD is the midsegment of the trapezoid WXYZ
From the properties of Midsegment of trapezoid we have :
0. The midsegment of a trapezoid is parallel to each base.
,
1. The length of the midsegment of a trapezoid is equal to half the sum of the lengths of its bases.
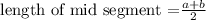
In the given figur, the mid segement CD= 22
length of parallel side is WZ=x+3
and the length of another side XY = 4x+1
so apply the mid segment length formula :
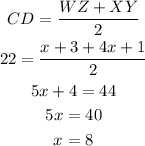
x=8,
For, XY :
Substitute x=8 into the given length expression of XY
XY =4x+1
XY=4(8)+1
XY=33
For, WZ :
Substitute x=8 into the given expression length of WZ
WZ=x+3
WZ=8+3
WZ=11
Answer :
a). x = 8
b). XY = 33
c). WZ = 11