To answer this question, we need to solve the equation for y in the third case:
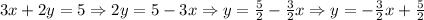
We can see from this case that for every value of x, there must be a value in y, and this is the main condition for a relationship to be a function. Then, y is a function of x.
In the fourth case, we have a similar case, for every possible value of x, there must be a value for y. Then, y is a function of x.
As we can see, the red graph is for the linear equation and the black one is for the one with the radical ( y = -sqrt(x+1)).
If we pass a vertical line to either function (alone), we will have only a point that passes through this vertical line, and with this graphical information, we can also say that both are functions of y (for each case).