Solution
The question gives us a graph that crosses the x-axis at 3 points: x = 1, x = 2, and x = -3. We are asked to find which of the factors on the graph is in the options given.
- Whenever a graph crosses the x-axis at a point "a", it implies that x = a is a root of the graph and as a result, (x - a) must be a factor of the graph.
- We can apply this to the question and derive the factors of the graph as follows:
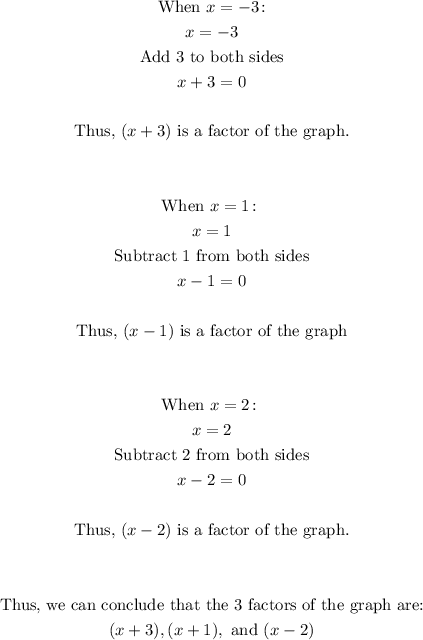
- Going through the options, we can see that only (x - 1) is present in the options.
- Thus, (x - 1) is the answer
Final Answer
(x - 1) is the answer (OPTION B)