Solution:
Given:
The right triangle can be sketched as shown below;
To get the distance between the building and the base of the ladder, we use the Pythagoras theorem since it is a right triangle.
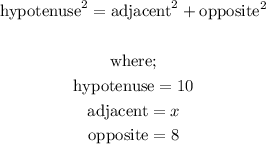
Hence,
![\begin{gathered} \text{hypotenuse}^2=\text{adjacent}^2+\text{opposite}^2 \\ 10^2=x^2+8^2 \\ 100=x^2+64 \\ 100-64=x^2 \\ 36=x^2 \\ x=\sqrt[]{36} \\ x=6 \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/emmxdoowv9twhw7k1795we02n4ilz03jgd.png)
Therefore, the distance between the building and the base of the ladder in feet is 6 feet.