Given three side lengths form an acute obtuse or a right triangle 17, 21, & 28
Check Right triangle

Not a Right triangle
An obtuse triangle is a triangle with one obtuse angle and two acute angles. Since a triangle's angles must sum to 180° in Euclidean geometry.
Not an Obtuse triangle
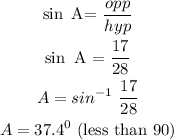

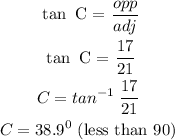
Hence it is acute angle because all angles are less than 90°