Question

Help I’ll give extra points!10. Camilla is saving to purchase a new pair of bowling shoes that will cost at least $39. She hasalready saved $19. What is the least amount of money she needs to save for the shoes?11. Suppose you earn $20 per hour working part time at a tax office. You want to earn at least$1,800 this month, before taxes. How many hours must you work?For problem 12, translate the phrase intoalgebraic inequality.12. A tour bus can seat 55 passengers. A minimum of 15 people must register for the tour to bookthe bus.

Answer 1

the least amount of money she needs to save is 20

the number of hours you must work is at least 90hours

Step-by-step explanation:

10) The pair of shoes cost at least $39

at least $39 means: the cost is ≥ 39

≥ means greater than or equal to

Amount saved = $19

Let the least amount of money = x

x + 19 ≥ 39

x ≥ 39-19

x ≥ 20

This means the least amount of money she needs to save is 20

11) Let the number of hours worked = x

Amount earned per hour = $20

Amount to be earned this month is at least $1,800

This means amount to be earned this month ≥ 1800

`\begin{gathered} 20* x\text{ }\ge\text{ 1800} \\ 20x\text{ }\ge\text{ 1800} \\ \text{Divide through by 20} \\ x\text{ }\ge\text{ }(1800)/(20) \\ x\text{ }\ge\text{ 90} \end{gathered}`

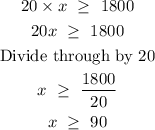
This means the number of hours you must work is at least 90hours