Question

Solve each system of the equation by elimination method. x+3y=-204x+5y=-38

Answer 1

Given the equation system:

`\begin{gathered} x+3y=-20 \\ 4x+5y=-38 \end{gathered}`

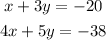
To solve this system using the elimination method, the first step is to multiply the first equation by 4 so that the leading coefficient is the same, i.e., both equations start with "4x"

`\begin{gathered} 4(x+3y=-20) \\ 4\cdot x+4\cdot3y=4\cdot(-20) \\ 4x+12y=-80 \end{gathered}`

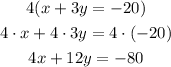
Then subtract the second equation from the first one

From the resulting expression, you can calculate the value of y

`\begin{gathered} 7y=-42 \\ (7y)/(7)=-(42)/(7) \\ y=-6 \end{gathered}`

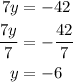
Next, you have to substitute the value of y in either the first or second equation to find the value of x:

`\begin{gathered} x+3y=-20 \\ x+3\cdot(-6)=-20 \\ x-18=-20 \\ x=-20+18 \\ x=-2 \end{gathered}`

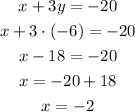
The solution of the system is (-2,-6)