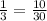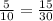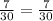Question

Rewrite all 3 fractions with the least common denominator between them.

Answer 1

To rewrite the given fraction with the least common denominator between them

The given fractions are,

`(1)/(3),(5)/(10),(7)/(30)`

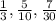
we get, LCM of 3,10,30 is 30

The equivalent fractions for the above factions as common denominator 30 is as follows,

For 1/3,

Multiply and divide by 10, we get,

`=(1)/(3)*(10)/(10)=(10)/(30)`

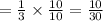
Using this we get,

`(1)/(3)=(10)/(30)`

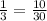
For 5/10,

Multiply and divide by 3, we get,

`(5)/(10)=(5)/(10)*(3)/(3)=(15)/(30)`

Using this we get,

`(5)/(10)=(15)/(30)`

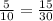
For 7/30,

Since the denominator 30, we get same fraction,

`(7)/(30)=(7)/(30)`

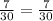
Answer is:

`(1)/(3)=(10)/(30)`

`(5)/(10)=(15)/(30)`

`(7)/(30)=(7)/(30)`