Since the function describing the height is a quadratic function with negative leading coefficient this means that this is a parabola that opens down. This also means that the maximum height will be given as the y component of the vertex of the parabola, then if we want to find the maximum height, we need to write the function in vertex form so let's do that:
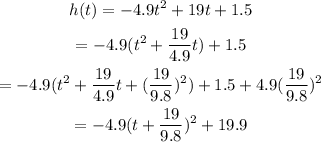
Hence the function can be written as:
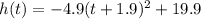
and its vertex is at (1.9,19.9) which means that the maximum height of the ball is 19.9 m