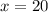Question

0.2x + 0.21x - 0.04 = 8.16Solve for "x".

Answer 1

Given the folllowing equation:

`0.2x+0.21x-0.04=8.16​`

You need to solve for "x" in order to find its value. To do this, you can follow the steps shown below:

1. You can apply the Addition property of equality by adding 0.04 to both sides of the equation:

`\begin{gathered} 0.2x+0.21x-0.04+(0.04)=8.16​+(0.04) \\ 0.2x+0.21x=8.2 \end{gathered}`

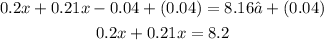
2. Now you need to add the like terms on the left side of the equation:

`0.41x=8.2`

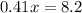
3. Finally, you can apply the Division property of equality by dividing both sides of the equation by 0.41:

`\begin{gathered} (0.41x)/(0.41)=(8.2)/(0.41) \\ \\ x=20 \end{gathered}`

The answer is:

`x=20`