We are given the following inequality
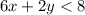
Let us first convert the inequality into slope-intercept form
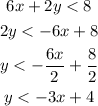
Comparing this inequality with the standard slope-intercept form we see that
Slope = -3 and y-intercept = 4
So the graph of the inequality is
The area left to the red line represents the solution of the inequality.
Now we need to check if the point (1, 1) lies left to the red line.
We can clearly see that point (1, 1) is just left to the red line hence it is a solution.
Therefore, it is true.